- (a)
- Write a linear function that will estimate the cost of driving a conventional Camry x miles, given the information above. (Hint: Think about what the units are for x and what the units should be for y. Then use the units of the information given to help you figure out what should be multiplied and what should be divided in order to give those desired units).
- (b)
- Write a linear function that will estimate the cost of driving a Hybrid Camry x miles, given the information above. (Hint: the function will be very similar to part(a)).
- (c)
- What are the “b-values” in these expressions? What do they represent in this context?
- (d)
- What are the “m-values” in these expressions (write them with their units)? What do these mean in this context?
- (e)
- What other factors could we be considering when comparing the “costs” between these two vehicles?
We explore how real-world applications of linear equations.
Your friends are trying to get an idea of how many people they can invite to their
wedding. The venue they’re looking at costs about $2,000 to rent and the event
coordinator suggested that they budget about $100 per attendee into the
costs.
- (a)
- Write a function that represents an estimate for how much this will cost if they have x attendees.
- (b)
- Now rewrite your function from part (a) with units for each of the values (including the variables). Do the units make sense? How would you check?
- (c)
- Suppose the venue has a maximum capacity of 250 people. What is an estimated maximum cost for using that venue?
- (d)
- Suppose your friends are trying to stick to about $20,000 for their budget. About how many guests can they invite?
- (a)
- Because the rate of change is constant at dollars per attendee, must be . The cost for attendees is so our value is this gives us the following equation: where is the total cost and is the number of attendees.
- (b)
- Substituting the units in we get the following: To check that the units make sense, we need to make sure that they match on both sides of the equation. The left side only has dollars, so the right side must match up. Since we are multiplying by attendees, the attendees will “cancel out.” This leaves us with only dollars on the right side, so both sides agree.
- (c)
- To find the cost for people we just have to plug in for .
The maximum cost is . - (d)
- Now we are given the total cost, so we can plug in for and find the
number of possible attendees.
The maximum amount of guests they can invite for $20000 would be 180 people.
As we can see, we can use in a variety of situations. These are not just points on a line or values in a table. In this context, they have a specific purpose and thinking about that context can help us understand linear modeling more deeply.
Now let’s
look closer at how we use for the previous example.
Now that we can see how to fit our information into a linear model, we can look at a
more challenging real world examples. Utility bills, and federal tax returns are both
great cases of tricky linear models. Take a look at the following example. - (a)
- What is the “b-value” in your equation? What does it mean in this context?
- (b)
- What is the “m-value” in your equation?
- (c)
- What are the units for the m-value? Explain why it makes sense that the “m-value” would have these units based on the x and y values’ units.
- (d)
- What does this m-value mean in this context?
- (a)
- The ”b-value” is 2000. This is also called the y-intercept, because it is the point that the line crosses the y-axis. In the context of this situation, it means that if 0 people attend the wedding the total cost will be $2000.
- (b)
- The “m-value” is 100. This is the slope or rate of change of the line.
- (c)
- In this context, it is $100 per attendee or . The units for x are attendees and the units for y are dollars. Since slope is defined by the change in y over the change in x, this makes sense that the units for are dollars per attendee.
- (d)
- In this context, it means that for each person that attends, the final cost will increase by $100.
Below
is some information for how electricity usage is billed in Columbus 
Reference: https://www.columbus.gov/Templates/Detail.aspx?id=2147500472
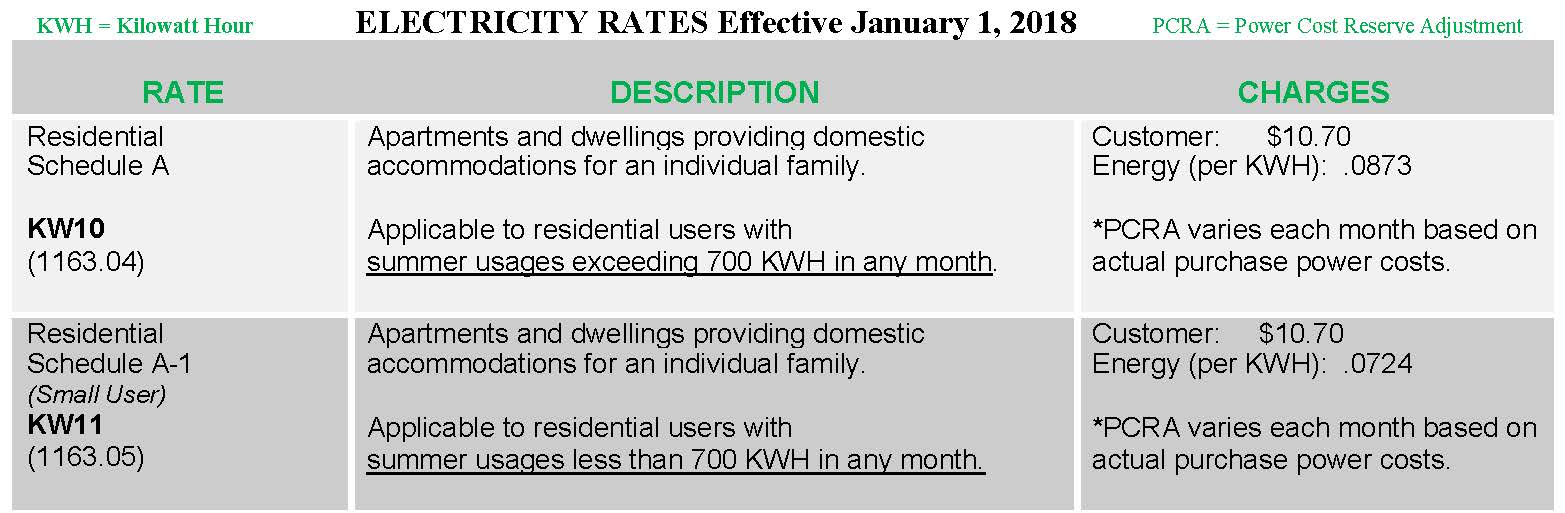
- (a)
- Write a function, or set of functions, which determine how much your electricity bill will be if you use x KWH in a month. Don’t worry about the variable PCRA rates. (Note: the 0.0873 and 0.0724 are dollar amounts, i.e. $0.0873 and $0.0724)
- (b)
- Suppose exactly 700 KWH are used in a month. Use Schedule A information to calculate a cost for the bill.
- (c)
- Suppose exactly 700 KWH are used in a month. Now use Schedule A-1 information to calculate a cost for the bill.
- (a)
- Unfortunately, we just have to know (assume) that the Energy (per KWH):
.0873 is taking about $ per KWH (that is what it means) If a household
is using less than 700 KWH, then the Schedule A-1 Instructions are used
for calculating their energy costs. So for KWH a month, we use: If a
household is using more than 700 KWH, then the Schedule A Instructions
are used for calculating their energy costs. So for x ¿ 700 KWH a month,
we use:
It does not seem clear which is used when x = 700 KWH (the wording does not really tell us) but using exactly 700 KWH hours is so rare that it doesnt matter.
- (b)
- represents KWH, and we want to know the cost for 700 KWH using Schedule A, so we plug into the Schedule A function:
- (c)
- represents KWH, and we want to know the cost for 700 KWH using Schedule A-1, so we plug into the Schedule A-1 function:
According to True Car (www.truecar.com), a 2018 Toyota Camry (conventional)
(29/41 MPG city/hwy) sells for an average of $22,030, and a Camry Hybrid (51/53
MPG city/hwy) sells for an average of $26,247. Currently gas prices are in the upper
$2 per gallon, so let’s estimate about $2.80 per gallon.