Local Extrema

$\newenvironment {prompt}{}{} \newcommand {\DeclareMathOperator }[2]{\@OldDeclareMathOperator {##1}{##2}\immediate \write \myfile {\unexpanded {\DeclareMathOperator }{\unexpanded {##1}}{\unexpanded {##2}}}} \newcommand {\reserved@a }[2]{} \newcommand {\HyperFirstAtBeginDocument }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{} \newcommand {\AppendGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Append \grfext@Check }{\grfext@Append \grfext@@Add }} \newcommand {\PrependGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Prepend \grfext@Check }{\grfext@Prepend \grfext@@Add }} \newcommand {\RemoveGraphicsExtensions }[1]{\@ifundefined {Gin@extensions}{\def \Gin@extensions {}}{\edef \grfext@tmp {\zap@space ##1 \@empty }\@for \grfext@ext :=\grfext@tmp \do {\def \grfext@next {\let \grfext@tmp \Gin@extensions \@expandtwoargs \@removeelement \grfext@ext \Gin@extensions \Gin@extensions \ifx \grfext@tmp \Gin@extensions \let \grfext@next \relax \fi \grfext@next }\grfext@next }}\grfext@Print \RemoveGraphicsExtensions } \newcommand {\epstopdfsetup }[0]{\setkeys {ETE}} \newcommand {\epstopdfcall }[1]{\ifETE@InsideSetfile \expandafter \@firstoftwo \else \expandafter \@secondoftwo \fi {‘##1}{\Gin@base \Gin@ext }} \newcommand {\epstopdfDeclareGraphicsRule }[4]{\ifx \\##4\\\@PackageError {epstopdf-base}{Conversion command is missing}\@ehc \else \begingroup \@ifundefined {Gin@rule@##1}{}{\@PackageInfo {epstopdf-base}{Redefining graphics rule for ‘##1’}}\endgroup \@namedef {Gin@rule@##1}####1{{##2}{##3}{\epstopdfcall {##4}}}\fi } \newcommand {\GetTitleStringSetup }[0]{\setkeys {gettitlestring}} \newcommand {\GetTitleString }[0]{\ifGTS@expand \expandafter \GetTitleStringExpand \else \expandafter \GetTitleStringNonExpand \fi } \newcommand {\GetTitleStringExpand }[1]{\def \GetTitleStringResult {##1}\begingroup \GTS@DisablePredefinedCmds \GTS@DisableHook \edef \x {\endgroup \noexpand \def \noexpand \GetTitleStringResult {\GetTitleStringResult }}\x } \newcommand {\GetTitleStringNonExpand }[1]{\def \GetTitleStringResult {##1}\global \let \GTS@GlobalString \GetTitleStringResult \begingroup \GTS@RemoveLeft \GTS@RemoveRight \endgroup \let \GetTitleStringResult \GTS@GlobalString } \newcommand {\GTS@DisableHook }[0]{} \newcommand {\label@hook }[0]{} \newcommand {\@currentlabelname }[0]{} \newcommand {\reserved@a }[0]{} \newcommand {\@safe@activestrue }[0]{} \newcommand {\@safe@activesfalse }[0]{} \newcommand {\nameref }[0]{\@ifstar \T@nameref \T@nameref } \newcommand {\Sectionformat }[2]{##1} \newcommand {\vnameref }[1]{\unskip ~\nameref {##1}\@vpageref [\unskip ]{##1}} \newcommand {\ref }[0]{\@ifstar \@refstar \T@ref } \newcommand {\pageref }[0]{\@ifstar \@pagerefstar \T@pageref } \newcommand {\nameref }[0]{\@ifstar \@namerefstar \T@nameref } \newcommand {\reserved@a }[2]{} \newcommand {\reserved@a }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{}$

Objectives:

1.: Be able to find and classify local extrema of functions of two variables.
2.: Understand why the second partials test makes sense geometrically.

Recap Video

The following video recaps the ideas of the section.

Test your understanding with the following questions.

Finding critical points of a function $f(x,y)$ means setting the gradient $\nabla f = \left \langle \answer {0}, \answer {0} \right \rangle .$

If $P$ is a critical point of $f(x,y)$ and $D(P) = f_{xx}(P)f_{yy}(P) - f_{xy}(P)^2 < 0$ , then $P$ is a .

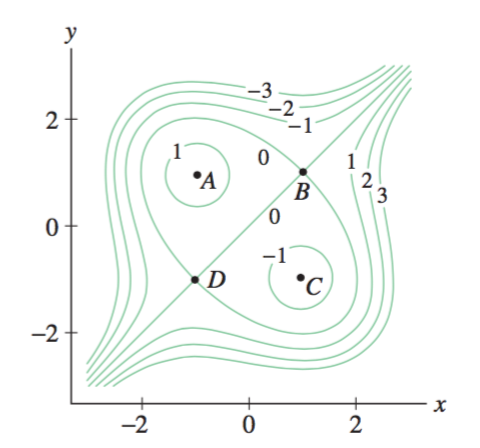
To recap:

The critical points of the function $f(x,y)$ happen where $\nabla f(x,y)= \vec {0}$ (or is undefined).
If is a critical point, we will classify it by looking at the second partials test. Let Then:
- If $D(a,b)> 0$ and $f_{xx}(a,b) > 0$ , then $(a,b)$ is a local minimum.
- If $D(a,b)>0$ and $f_{xx}(a,b)<0$ , then $(a,b)$ is a local maximum.
- If $D(a,b)<0$ , then $(a,b)$ is a saddle point.

Example

You can watch the following video which works out an example of finding local extrema.

Find and classify the local extrema of $f(x,y) = 2x^3+9xy^2+15x^2+27y^2$ .

Problems

Find and classify the critical points of the function $f(x,y) = 2x^2+4xy+3y^2-4x-6y$ .

Steps:

The gradient of the function is $\nabla f(x,y) = \left \langle \answer {4x+4y-4},\answer {4x+6y-6} \right \rangle$ .
Setting $\nabla f(x,y) = \left \langle 0,0 \right \rangle$ and solving for $x$ and $y$ gives one critical point, namely: $(\answer {0},\answer {1})$ .
The number $D$ from the second part of the proposition above is equal to $D = \answer {8}$
Since $D$
$0$ and $f_{xx} = \answer {4}$ we get that the critical point is a:

Consider $f(x,y) = x^3+y^3-3x-12y+1$ . Find and classify all local extrema of the function.

Steps:

The gradient $\nabla f = \left \langle \answer {3x^2-3},\answer {3y^2-12}\right \rangle$ .
Setting $\nabla f(x,y) = \left \langle 0,0 \right \rangle$ gives the equations $3x^2-3 = 0$ $3y^2-12 = 0.$
Solving the first equation for $x$ gives $x = \pm \answer {1}$ . Solving the second equation gives $y = \pm \answer {2}$ .
Together this gives how many critical points? $\answer {4}$ .
For both $(1,-2)$ and $(-1,2)$ , we get $D$
$0$ , which means these two points are
.
For $(-1,-2)$ , we get $D(-1,-2)$
$0$ and $f_{xx}(-1,-2)$
$0$ , which means $(-1,-2)$ is a
.
For $(1,2)$ , we get $D(1,2)$
$0$ and $f_{xx}(1,2)$
$0$ , which means $(-1,-2)$ is a
.

The function $f(x,y) = x^3-y^3+6xy$ has how many critical points? $\answer {2}$ .

The two points are $(2,\answer {-2})$ and $(0,\answer {0})$ .

The point $(2,-2)$ is a and the point $(0,0)$ is a .

The function $f(x,y) = xy-2x-2y-x^2-y^2$ has how many critical points? $\answer {1}$ .

The critical point is at $(\answer {-2},\answer {-2})$ .

The point $(-2,-2)$ is a .

The function $f(x,y) = e^{-x^2-y^2}$ has only one critical point at $(\answer {0},\answer {0})$ , and it classifies as a .

The function $f(x,y) = \frac {2}{3}x^3+2xy^2-y^3-50x$ has how many critical points? $\answer {4}$

There are how many of each of the following?

Local minima: $\answer {1}$
Local maxima: $\answer {1}$
Saddle points: $\answer {2}$

Consider the contour map of the function $f(x,y)$ given in the figure.

Figure 1: Taken from Rogawski’s Calculus.

Each of the points $A,B,C,D$ is a critical point for $f(x,y)$ . Classify them as a local maximum, minimum, or saddle point.

The point $A$ is a
.
The point $B$ is a
.
The point $C$ is a
.
The point $D$ is a
.

Press...	...to do
left/right arrows	Move cursor
shift+left/right arrows	Select region
ctrl+a	Select all
ctrl+x/c/v	Cut/copy/paste
ctrl+z/y	Undo/redo
ctrl+left/right	Add entry to list or column to matrix
shift+ctrl+left/right	Add copy of current entry/column to to list/matrix
ctrl+up/down	Add row to matrix
shift+ctrl+up/down	Add copy of current row to matrix
ctrl+backspace	Delete current entry in list or column in matrix
ctrl+shift+backspace	Delete current row in matrix

Type...	...to get
norm	$\|\|\blue{[?]}\|\|$
text	$\text{\blue{[?]}}$
sym_name	$\backslash\texttt{\blue{[?]}}$
abs	$\left\|\blue{[?]}\right\|$
sqrt	$\sqrt{\blue{[?]}}$
paren	$\left(\blue{[?]}\right)$
floor	$\lfloor \blue{[?]} \rfloor$
factorial	$\blue{[?]}!$
exp	${\blue{[?]}}^{\blue{[?]}}$
sub	${\blue{[?]}}_{\blue{[?]}}$
frac	$\dfrac{\blue{[?]}}{\blue{[?]}}$
int	$\displaystyle\int{\blue{[?]}}d\blue{[?]}$
defi	$\displaystyle\int_{\blue{[?]}}^{\blue{[?]}}\blue{[?]}d\blue{[?]}$
deriv	$\displaystyle\frac{d}{d\blue{[?]}}\blue{[?]}$
sum	$\displaystyle\sum_{\blue{[?]}}^{\blue{[?]}}\blue{[?]}$
prod	$\displaystyle\prod_{\blue{[?]}}^{\blue{[?]}}\blue{[?]}$
root	$\sqrt[\blue{[?]}]{\blue{[?]}}$
vec	$\left\langle \blue{[?]} \right\rangle$
mat	$\left(\begin{matrix} \blue{[?]} \end{matrix}\right)$
*	$\cdot$
infinity	$\infty$
arcsin	$\arcsin\left(\blue{[?]}\right)$
arccos	$\arccos\left(\blue{[?]}\right)$
arctan	$\arctan\left(\blue{[?]}\right)$
sin	$\sin\left(\blue{[?]}\right)$
cos	$\cos\left(\blue{[?]}\right)$
tan	$\tan\left(\blue{[?]}\right)$
sec	$\sec\left(\blue{[?]}\right)$
csc	$\csc\left(\blue{[?]}\right)$
cot	$\cot\left(\blue{[?]}\right)$
log	$\log\left(\blue{[?]}\right)$
ln	$\ln\left(\blue{[?]}\right)$
alpha	$\alpha$
beta	$\beta$
gamma	$\gamma$
delta	$\delta$
epsilon	$\epsilon$
zeta	$\zeta$
eta	$\eta$
theta	$\theta$
iota	$\iota$
kappa	$\kappa$
lambda	$\lambda$
mu	$\mu$
nu	$\nu$
xi	$\xi$
omicron	$\omicron$
pi	$\pi$
rho	$\rho$
sigma	$\sigma$
tau	$\tau$
upsilon	$\upsilon$
phi	$\phi$
chi	$\chi$
psi	$\psi$
omega	$\omega$
Gamma	$\Gamma$
Delta	$\Delta$
Theta	$\Theta$
Lambda	$\Lambda$
Xi	$\Xi$
Pi	$\Pi$
Sigma	$\Sigma$
Phi	$\Phi$
Psi	$\Psi$
Omega	$\Omega$

Recap Video

Example

Problems

Controls

Symbols

Settings