Exploring the Gradient Properties

$\newenvironment {prompt}{}{} \newcommand {\DeclareMathOperator }[2]{\@OldDeclareMathOperator {##1}{##2}\immediate \write \myfile {\unexpanded {\DeclareMathOperator }{\unexpanded {##1}}{\unexpanded {##2}}}} \newcommand {\reserved@a }[2]{} \newcommand {\HyperFirstAtBeginDocument }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{} \newcommand {\AppendGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Append \grfext@Check }{\grfext@Append \grfext@@Add }} \newcommand {\PrependGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Prepend \grfext@Check }{\grfext@Prepend \grfext@@Add }} \newcommand {\RemoveGraphicsExtensions }[1]{\@ifundefined {Gin@extensions}{\def \Gin@extensions {}}{\edef \grfext@tmp {\zap@space ##1 \@empty }\@for \grfext@ext :=\grfext@tmp \do {\def \grfext@next {\let \grfext@tmp \Gin@extensions \@expandtwoargs \@removeelement \grfext@ext \Gin@extensions \Gin@extensions \ifx \grfext@tmp \Gin@extensions \let \grfext@next \relax \fi \grfext@next }\grfext@next }}\grfext@Print \RemoveGraphicsExtensions } \newcommand {\epstopdfsetup }[0]{\setkeys {ETE}} \newcommand {\epstopdfcall }[1]{\ifETE@InsideSetfile \expandafter \@firstoftwo \else \expandafter \@secondoftwo \fi {‘##1}{\Gin@base \Gin@ext }} \newcommand {\epstopdfDeclareGraphicsRule }[4]{\ifx \\##4\\\@PackageError {epstopdf-base}{Conversion command is missing}\@ehc \else \begingroup \@ifundefined {Gin@rule@##1}{}{\@PackageInfo {epstopdf-base}{Redefining graphics rule for ‘##1’}}\endgroup \@namedef {Gin@rule@##1}####1{{##2}{##3}{\epstopdfcall {##4}}}\fi } \newcommand {\GetTitleStringSetup }[0]{\setkeys {gettitlestring}} \newcommand {\GetTitleString }[0]{\ifGTS@expand \expandafter \GetTitleStringExpand \else \expandafter \GetTitleStringNonExpand \fi } \newcommand {\GetTitleStringExpand }[1]{\def \GetTitleStringResult {##1}\begingroup \GTS@DisablePredefinedCmds \GTS@DisableHook \edef \x {\endgroup \noexpand \def \noexpand \GetTitleStringResult {\GetTitleStringResult }}\x } \newcommand {\GetTitleStringNonExpand }[1]{\def \GetTitleStringResult {##1}\global \let \GTS@GlobalString \GetTitleStringResult \begingroup \GTS@RemoveLeft \GTS@RemoveRight \endgroup \let \GetTitleStringResult \GTS@GlobalString } \newcommand {\GTS@DisableHook }[0]{} \newcommand {\label@hook }[0]{} \newcommand {\@currentlabelname }[0]{} \newcommand {\reserved@a }[0]{} \newcommand {\@safe@activestrue }[0]{} \newcommand {\@safe@activesfalse }[0]{} \newcommand {\nameref }[0]{\@ifstar \T@nameref \T@nameref } \newcommand {\Sectionformat }[2]{##1} \newcommand {\vnameref }[1]{\unskip ~\nameref {##1}\@vpageref [\unskip ]{##1}} \newcommand {\ref }[0]{\@ifstar \@refstar \T@ref } \newcommand {\pageref }[0]{\@ifstar \@pagerefstar \T@pageref } \newcommand {\nameref }[0]{\@ifstar \@namerefstar \T@nameref } \newcommand {\reserved@a }[2]{} \newcommand {\reserved@a }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{}$

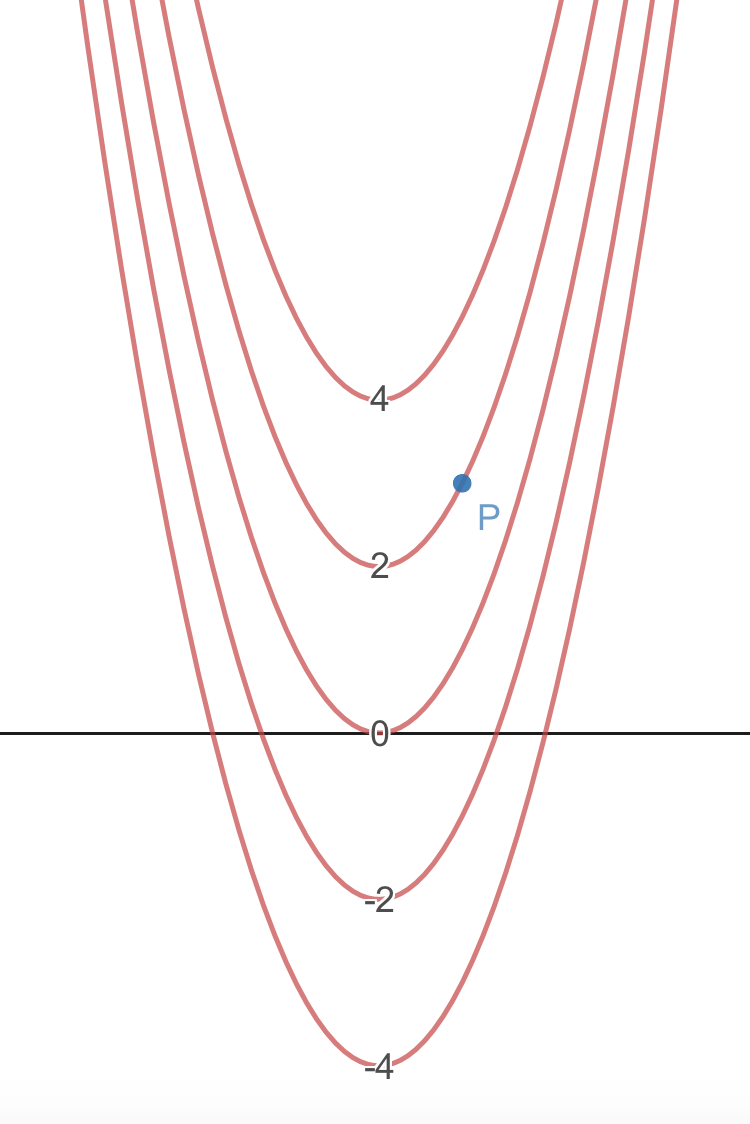
Gradient is Perpendicular to Level Curves

The following is a contour map for a function $z = f(x,y)$ . We will examine why at any point $P(a,b)$ , the gradient $\nabla f(P)$ has to be perpendicular to the (tangent to the) level curve.

Everything below refers to this contour map. We can parametrize the level curve passing through $P$ , call it $\textbf {r}(t)$ . Assume $P$ corresponds to $t=0$ .

Since $\textbf {r}(t)$ represents the level curve through $P$ , we know that $f(\textbf {r}(t))$ is constant, which means $f(\textbf {r}(t)) = \answer {2}.$
Since $f(\textbf {r}(t))$ is constant, we know $\frac {d}{dt}f(\textbf {r}(t)) = \answer {0}$ .
By the chain rule, $\frac {d}{dt}f(\textbf {r}(t)) = \nabla f(\textbf {r}(t)) \cdot \textbf {r}'(t).$
The point $P$ corresponds to $t=0$ , so we can plug in $t=0$ to get $\nabla f(P) \cdot \textbf {r}'(0) = 0.$
What does $\textbf {r}'(0)$ represent?
Since the dot product is $0$ , we know that $\nabla f(P)$ and $\textbf {r}'(0)$ are
.

Therefore, $\nabla f(P)$ is perpendicular to the tangent to the level curve.

Gradient Points in the Direction of Maximum Increase

Now we will see why the gradient points in the direction of maximum increase. This means of all vectors $\textbf {u}$ , the one which gives a maximum value of $D_{\textbf {u}}f(P)$ is $\textbf {u} = \nabla f(P)$ . It all comes down to the formula $D_{\textbf {u}}f(P) = \nabla f(P) \cdot \hat {\textbf {u}}.$

By the cosine formula for the dot product, which of the following is equal to $D_{\textbf {u}}f(P)$ ?
Since $0 \leq \theta \leq \pi$ , the maximum value of $D_{\textbf {u}}f(P)$ happens when $\theta = \answer {0}$ .
This means that $\nabla f(P)$ and $\textbf {u}$ have what relationship?

Therefore, the maximum rate of change happens in the direction of $\nabla f(P)$ .

The Magnitude of the Gradient Is the Maximum Rate of Increase

The last thing we will consider is why $\| \nabla f(P) \|$ is the maximum rate of increase at the point $P$ . Again it boils down to $D_{\textbf {u}}f(P) = \nabla f(P) \cdot \hat {\textbf {u}} = \| \nabla f(P) \| \cos (\theta ).$ We saw in the previous section that the maximum value of $D_{\textbf {u}}f(P)$ happens when $\theta = \answer {0}$ , or when $\textbf {u}$ points in the same direction as $\nabla f(P)$ . If $\theta = 0$ , then $\cos (\theta ) = \answer {1}$ , so in this case, $D_{\textbf {u}}f(P) = \| \nabla f(P) \| \cos (0) = \| \nabla f(P)\| ,$ which is exactly what we wanted.

Press...	...to do
left/right arrows	Move cursor
shift+left/right arrows	Select region
ctrl+a	Select all
ctrl+x/c/v	Cut/copy/paste
ctrl+z/y	Undo/redo
ctrl+left/right	Add entry to list or column to matrix
shift+ctrl+left/right	Add copy of current entry/column to to list/matrix
ctrl+up/down	Add row to matrix
shift+ctrl+up/down	Add copy of current row to matrix
ctrl+backspace	Delete current entry in list or column in matrix
ctrl+shift+backspace	Delete current row in matrix

Type...	...to get
norm	$\|\|\blue{[?]}\|\|$
text	$\text{\blue{[?]}}$
sym_name	$\backslash\texttt{\blue{[?]}}$
abs	$\left\|\blue{[?]}\right\|$
sqrt	$\sqrt{\blue{[?]}}$
paren	$\left(\blue{[?]}\right)$
floor	$\lfloor \blue{[?]} \rfloor$
factorial	$\blue{[?]}!$
exp	${\blue{[?]}}^{\blue{[?]}}$
sub	${\blue{[?]}}_{\blue{[?]}}$
frac	$\dfrac{\blue{[?]}}{\blue{[?]}}$
int	$\displaystyle\int{\blue{[?]}}d\blue{[?]}$
defi	$\displaystyle\int_{\blue{[?]}}^{\blue{[?]}}\blue{[?]}d\blue{[?]}$
deriv	$\displaystyle\frac{d}{d\blue{[?]}}\blue{[?]}$
sum	$\displaystyle\sum_{\blue{[?]}}^{\blue{[?]}}\blue{[?]}$
prod	$\displaystyle\prod_{\blue{[?]}}^{\blue{[?]}}\blue{[?]}$
root	$\sqrt[\blue{[?]}]{\blue{[?]}}$
vec	$\left\langle \blue{[?]} \right\rangle$
mat	$\left(\begin{matrix} \blue{[?]} \end{matrix}\right)$
*	$\cdot$
infinity	$\infty$
arcsin	$\arcsin\left(\blue{[?]}\right)$
arccos	$\arccos\left(\blue{[?]}\right)$
arctan	$\arctan\left(\blue{[?]}\right)$
sin	$\sin\left(\blue{[?]}\right)$
cos	$\cos\left(\blue{[?]}\right)$
tan	$\tan\left(\blue{[?]}\right)$
sec	$\sec\left(\blue{[?]}\right)$
csc	$\csc\left(\blue{[?]}\right)$
cot	$\cot\left(\blue{[?]}\right)$
log	$\log\left(\blue{[?]}\right)$
ln	$\ln\left(\blue{[?]}\right)$
alpha	$\alpha$
beta	$\beta$
gamma	$\gamma$
delta	$\delta$
epsilon	$\epsilon$
zeta	$\zeta$
eta	$\eta$
theta	$\theta$
iota	$\iota$
kappa	$\kappa$
lambda	$\lambda$
mu	$\mu$
nu	$\nu$
xi	$\xi$
omicron	$\omicron$
pi	$\pi$
rho	$\rho$
sigma	$\sigma$
tau	$\tau$
upsilon	$\upsilon$
phi	$\phi$
chi	$\chi$
psi	$\psi$
omega	$\omega$
Gamma	$\Gamma$
Delta	$\Delta$
Theta	$\Theta$
Lambda	$\Lambda$
Xi	$\Xi$
Pi	$\Pi$
Sigma	$\Sigma$
Phi	$\Phi$
Psi	$\Psi$
Omega	$\Omega$

Gradient is Perpendicular to Level Curves

Gradient Points in the Direction of Maximum Increase

The Magnitude of the Gradient Is the Maximum Rate of Increase

Controls

Symbols

Settings