Reflection Coefficient

$\newenvironment {prompt}{}{} \newcommand {\ungraded }[0]{} \newcommand {\todo }[0]{} \newcommand {\oiint }[0]{{\large \bigcirc }\kern -1.56em\iint } \newcommand {\mooculus }[0]{\textsf {\textbf {MOOC}\textnormal {\textsf {ULUS}}}} \newcommand {\npnoround }[0]{\nprounddigits {-1}} \newcommand {\npnoroundexp }[0]{\nproundexpdigits {-1}} \newcommand {\npunitcommand }[1]{\ensuremath {\mathrm {#1}}} \newcommand {\RR }[0]{\mathbb R} \newcommand {\R }[0]{\mathbb R} \newcommand {\N }[0]{\mathbb N} \newcommand {\Z }[0]{\mathbb Z} \newcommand {\sagemath }[0]{\textsf {SageMath}} \newcommand {\d }[0]{\mathop {}\!d} \newcommand {\l }[0]{\ell } \newcommand {\ddx }[0]{\frac {d}{\d x}} \newcommand {\zeroOverZero }[0]{\ensuremath {\boldsymbol {\tfrac {0}{0}}}} \newcommand {\inftyOverInfty }[0]{\ensuremath {\boldsymbol {\tfrac {\infty }{\infty }}}} \newcommand {\zeroOverInfty }[0]{\ensuremath {\boldsymbol {\tfrac {0}{\infty }}}} \newcommand {\zeroTimesInfty }[0]{\ensuremath {\small \boldsymbol {0\cdot \infty }}} \newcommand {\inftyMinusInfty }[0]{\ensuremath {\small \boldsymbol {\infty -\infty }}} \newcommand {\oneToInfty }[0]{\ensuremath {\boldsymbol {1^\infty }}} \newcommand {\zeroToZero }[0]{\ensuremath {\boldsymbol {0^0}}} \newcommand {\inftyToZero }[0]{\ensuremath {\boldsymbol {\infty ^0}}} \newcommand {\numOverZero }[0]{\ensuremath {\boldsymbol {\tfrac {\#}{0}}}} \newcommand {\dfn }[0]{\textbf } \newcommand {\unit }[0]{\mathop {}\!\mathrm } \newcommand {\eval }[1]{\bigg [ #1 \bigg ]} \newcommand {\seq }[1]{\left ( #1 \right )} \newcommand {\epsilon }[0]{\varepsilon } \newcommand {\phi }[0]{\varphi } \newcommand {\iff }[0]{\Leftrightarrow } \DeclareMathOperator {\arccot }{arccot} \DeclareMathOperator {\arcsec }{arcsec} \DeclareMathOperator {\arccsc }{arccsc} \DeclareMathOperator {\si }{Si} \DeclareMathOperator {\scal }{scal} \DeclareMathOperator {\sign }{sign} \newcommand {\arrowvec }[1]{{\overset {\rightharpoonup }{#1}}} \newcommand {\vec }[1]{{\overset {\boldsymbol {\rightharpoonup }}{\mathbf {#1}}}\hspace {0in}} \newcommand {\point }[1]{\left (#1\right )} \newcommand {\pt }[1]{\mathbf {#1}} \newcommand {\Lim }[2]{\lim _{\point {#1} \to \point {#2}}} \DeclareMathOperator {\proj }{\mathbf {proj}} \newcommand {\veci }[0]{{\boldsymbol {\hat {\imath }}}} \newcommand {\vecj }[0]{{\boldsymbol {\hat {\jmath }}}} \newcommand {\veck }[0]{{\boldsymbol {\hat {k}}}} \newcommand {\vecl }[0]{\vec {\boldsymbol {\l }}} \newcommand {\uvec }[1]{\mathbf {\hat {#1}}} \newcommand {\utan }[0]{\mathbf {\hat {t}}} \newcommand {\unormal }[0]{\mathbf {\hat {n}}} \newcommand {\ubinormal }[0]{\mathbf {\hat {b}}} \newcommand {\dotp }[0]{\bullet } \newcommand {\cross }[0]{\boldsymbol \times } \newcommand {\grad }[0]{\boldsymbol \nabla } \newcommand {\divergence }[0]{\grad \dotp } \newcommand {\curl }[0]{\grad \cross } \newcommand {\lto }[0]{\mathop {\longrightarrow \,}\limits } \newcommand {\bar }[0]{\overline } \newcommand {\surfaceColor }[0]{violet} \newcommand {\surfaceColorTwo }[0]{redyellow} \newcommand {\sliceColor }[0]{greenyellow} \newcommand {\vector }[1]{\left \langle #1\right \rangle } \newcommand {\sectionOutcomes }[0]{} \newcommand {\HyperFirstAtBeginDocument }[0]{\AtBeginDocument }$

In this section, we will derive the equation for the reflection coefficient. The reflection coefficient relates the forward-going voltage with reflected voltage.

Reflection coefficient at the load

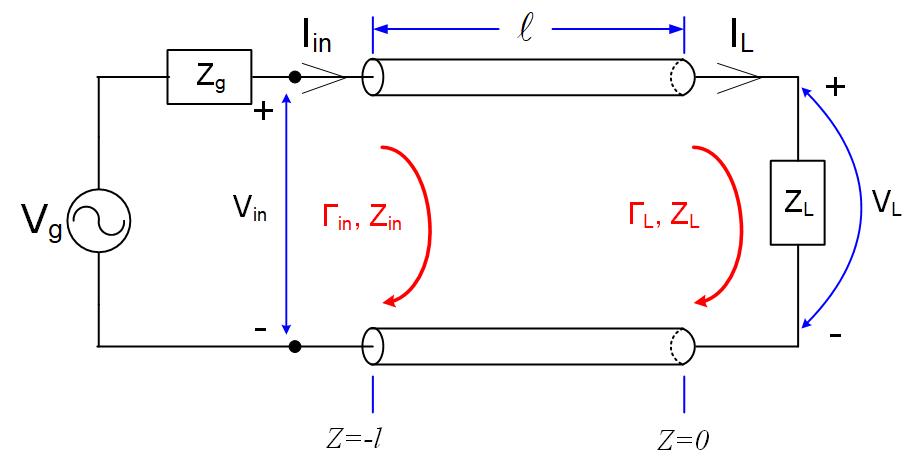
Equations eq:RCvtl-eq:RCctl represent the voltage and current on a lossless transmission line shown in Figure fig:RCTLCircuit.

$\begin{eqnarray} \tilde{V}(z)=\tilde{V}_0^+ e^{-j \beta z} +\tilde{V}_0^- e^{j \beta z} \label{eq:RCvtl} \\ I(z)=\frac{\tilde{V}_0^+}{Z_0} e^{- j \beta z} - \frac{\tilde{V}_0^-}{Z_0} e^{j \beta z}\label{eq:RCctl} \end{eqnarray}$

Figure 1: Transmission Line connects generator and the load.

We set up the z-axis so that the $z=0$ is at the load, and the generator is at $z=-l$ . At $z=0$ , the load impedance is connected. The definition of impedance is $Z=V/I$ , therefore at the z=0 end of the transmission line, the voltage and current on the transmission line at that point have to obey boundary condition that the load impedance imposes.

$\begin{eqnarray} Z_L=\frac{V(0)}{I(0)} \nonumber \end{eqnarray}$

Substituting z=0, the boundary condition, in Equations eq:RCvtl-eq:RCctl, we get Equations eq:RCvtl1-eq:RCctl1.

$\begin{eqnarray} \tilde{V}(0)=\tilde{V}_0^+ e^{-j \beta 0} +\tilde{V}_0^- e^{j \beta 0} = \tilde{V}_0^+ + \tilde{V}_0^- \label{eq:RCvtl1} \\ I(0)=\frac{\tilde{V}_0^+}{Z_0} e^{- j \beta 0} - \frac{\tilde{V}_0^-}{Z_0} e^{ j \beta 0} =\frac{\tilde{V}_0^+}{Z_0} - \frac{\tilde{V}_0^-}{Z_0}\label{eq:RCctl1} \end{eqnarray}$

Dividing the two above equations, we get the impedance at the load.

$\begin{eqnarray} Z_L=Z_0 \frac{\tilde{V}_0^+ + \tilde{V}_0^-}{\tilde{V}_0^+ - \tilde{V}_0^-} \end{eqnarray}$

We can now solve the above equation for $\tilde {V}_0^-$

$\begin{eqnarray} \frac{Z_L}{Z_0} (\tilde{V}_0^+ - \tilde{V}_0^-) = \tilde{V}_0^+ + \tilde{V}_0^- \nonumber \\ (\frac{Z_L}{Z_0}-1)\tilde{V}_0^+ =(\frac{Z_L}{Z_0}+1) \tilde{V}_0^- \nonumber \\ \frac{\tilde{V}_0^-}{\tilde{V}_0^+} = \frac{\frac{Z_L}{Z_0}-1 }{ \frac{Z_L}{Z_0}+1 } = \frac{z_L-1}{z_L+1} \nonumber \\ \Gamma_L=\frac{\tilde{V}_0^-}{\tilde{V}_0^+} = \frac{Z_L -Z_0}{Z_L +Z_0} \end{eqnarray}$

Lowercase $z_L$ is called the ”normalized load impedance”. It is the actual impedance divided by the transmission line impedance $z_L=\frac {Z_L}{Z_0}$ . For example, if the load impedance is $Z_L=100 \Omega$ , and the transmission-line impedance is $Z)=50 \Omega$ , then the normalized impedance is $z_L=\frac {100 \Omega }{50 \Omega }=2$ . Normalized impedance is a unitless quantity.

$\Gamma _L=\frac {\tilde {V}_0^-}{\tilde {V}_0^+}= \frac {Z_L -Z_0}{Z_L +Z_0}$ is the voltage reflection coefficient at the load. $\Gamma _L$ relates the reflected and incident voltage phasor and the load $Z_L$ and transmission line impedance $Z_0$ . The voltage reflection coefficient at the load is, in general, a complex number, it has a magnitude and a phase $\Gamma _L=|\Gamma _L| e^{j \angle \Gamma _L}$ .

Example

(a): 100 $\Omega$ transmission line is terminated in a series connection of a 50 $\Omega$ resistor and 10 pF capacitor. The frequency of operation is 100 MHz. Find the voltage reflection coefficient.
(b): For purely reactive load $Z_L=j 50 \Omega$ , find the reflection coefficient.

Voltage and Current on a transmission line

Now that we related forward and reflected voltage on a transmission line with the reflection coefficient at the load, we can re-write the equations for the current and voltage on a transmission line as:

$\begin{eqnarray} \tilde{V}(z)= \tilde{V}_0^+ (e^{-j \beta z} + \Gamma_L e^{j \beta z } ) \label{eq:vtlfin} \\ I(z)= \frac{\tilde{V}_0^+}{Z_0} (e^{-j \beta z} - \Gamma e^{j \beta z} ) \label{eq:itlfin} \end{eqnarray}$

We see that if we know the length of the line, line type, the load impedance, and the transmission line impedance, we can calculate all variables above, except for $\tilde {V}_0^+$ . In the following chapters, we will derive the equation for the forward going voltage at the load, but first, we will look at little more at the various reflection coefficients on a transmission line.

Reflection coefficient anywhere on the line

Equations eq:RCvtl-eq:RCctl can be concisely written as

$\begin{eqnarray} \tilde{V}(z) =\tilde{V}(z)^+ + \tilde{V}(z)^- \\ \tilde{I}(z) =\tilde{I}(z)^+ + \tilde{I}(z)^- \end{eqnarray}$

Where $\tilde {V}(z)^+$ is the forward voltage anywhere on the line, $\tilde {V}(z)^-$ is reflected voltage anywhere on the line, $\tilde {I}(z)^+$ is the forward current anywhere on the line, and $\tilde {I}(z)^-$ is the reflected current anywhere on the line.

We can then define a reflection coefficient anywhere on the line as

$\Gamma (z)=\frac {\tilde {V}(z)^-}{\tilde {V}(z)^+} = \frac {\tilde {V}_0^- e^{j \beta z}}{\tilde {V}_0^+ e^{-j \beta z}} = \frac {\tilde {V}_0^-}{\tilde {V}_0^+} e^{2j \beta z}$ is a voltage reflection coefficient anywhere on the line. $\Gamma (z)$ relates the reflected and incident voltage phasor at any z.

Since we already defined $\Gamma _L=\frac {\tilde {V}_0^-}{\tilde {V}_0^+}$ as the reflection coefficient at the load, we can now simplify the general reflection coefficient as

$\begin{equation} \Gamma(z)=\Gamma_L e^{2j \beta z} \end{equation}$

It is important to remember that we defined points between the generator and the load as the negative z-axis. If the line length is, for example, l m long, the generator is then at z=-l m, and the load at z=0. To find the reflection coefficient at some distance $l/2$ m away from the load, at $z=-l/2$ m, the equation for the reflection coefficient will be

$\begin{equation} \Gamma(z=-l/2)=\Gamma_L e^{-2j \beta l/2} \end{equation}$

Since we already defined the reflection coefficient at the load, the reflection at any point on the line $z=-l$ is

$\begin{eqnarray} \Gamma(z=-l)=\Gamma_L e^{-2j \beta l} \\ \Gamma(z=-l)=|\Gamma_L| e^{j ( \angle \Gamma_L - 2 \beta l) } \end{eqnarray}$

Reflection coefficient at the input of the transmission line

Using the reasoning above, the reflection coefficient at the input of the line whose length is $l$ is

$\begin{equation} \Gamma(z=-l)=\Gamma_{in}=\Gamma_L e^{-2j \beta l} \end{equation}$

The reflection coefficient at the load is $\Gamma _L=0.5 e^{j60^0}$ . Find the input reflection coefficient if the electrical length of the line is $\beta l= 45^0$ .

The reflection coefficient at the input of the line is $\Gamma (z=-l)=\Gamma _{in}=|\Gamma _L| e^{j ( \angle \Gamma _L - 2 \beta l) }$ .

We substitute the expression for $\Gamma _L=0.5 e^{j60^0}$ and $\beta l= 90^0$ , we get the reflection coefficient at the input of the line $\Gamma _{in}=0.5 e^{-j30 }$ .