Autonomous Differential Equations

$\newenvironment {prompt}{}{} \newcommand {\DeclareMathOperator }[2]{\@OldDeclareMathOperator {##1}{##2}\immediate \write \myfile {\unexpanded {\DeclareMathOperator }{\unexpanded {##1}}{\unexpanded {##2}}}} \newcommand {\reserved@a }[2]{} \newcommand {\HyperFirstAtBeginDocument }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{} \newcommand {\AppendGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Append \grfext@Check }{\grfext@Append \grfext@@Add }} \newcommand {\PrependGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Prepend \grfext@Check }{\grfext@Prepend \grfext@@Add }} \newcommand {\RemoveGraphicsExtensions }[1]{\@ifundefined {Gin@extensions}{\def \Gin@extensions {}}{\edef \grfext@tmp {\zap@space ##1 \@empty }\@for \grfext@ext :=\grfext@tmp \do {\def \grfext@next {\let \grfext@tmp \Gin@extensions \@expandtwoargs \@removeelement \grfext@ext \Gin@extensions \Gin@extensions \ifx \grfext@tmp \Gin@extensions \let \grfext@next \relax \fi \grfext@next }\grfext@next }}\grfext@Print \RemoveGraphicsExtensions } \newcommand {\epstopdfsetup }[0]{\setkeys {ETE}} \newcommand {\epstopdfcall }[1]{\ifETE@InsideSetfile \expandafter \@firstoftwo \else \expandafter \@secondoftwo \fi {‘##1}{\Gin@base \Gin@ext }} \newcommand {\epstopdfDeclareGraphicsRule }[4]{\ifx \\##4\\\@PackageError {epstopdf-base}{Conversion command is missing}\@ehc \else \begingroup \@ifundefined {Gin@rule@##1}{}{\@PackageInfo {epstopdf-base}{Redefining graphics rule for ‘##1’}}\endgroup \@namedef {Gin@rule@##1}####1{{##2}{##3}{\epstopdfcall {##4}}}\fi } \newcommand {\GetTitleStringSetup }[0]{\setkeys {gettitlestring}} \newcommand {\GetTitleString }[0]{\ifGTS@expand \expandafter \GetTitleStringExpand \else \expandafter \GetTitleStringNonExpand \fi } \newcommand {\GetTitleStringExpand }[1]{\def \GetTitleStringResult {##1}\begingroup \GTS@DisablePredefinedCmds \GTS@DisableHook \edef \x {\endgroup \noexpand \def \noexpand \GetTitleStringResult {\GetTitleStringResult }}\x } \newcommand {\GetTitleStringNonExpand }[1]{\def \GetTitleStringResult {##1}\global \let \GTS@GlobalString \GetTitleStringResult \begingroup \GTS@RemoveLeft \GTS@RemoveRight \endgroup \let \GetTitleStringResult \GTS@GlobalString } \newcommand {\GTS@DisableHook }[0]{} \newcommand {\label@hook }[0]{} \newcommand {\@currentlabelname }[0]{} \newcommand {\reserved@a }[0]{} \newcommand {\@safe@activestrue }[0]{} \newcommand {\@safe@activesfalse }[0]{} \newcommand {\nameref }[0]{\@ifstar \T@nameref \T@nameref } \newcommand {\Sectionformat }[2]{##1} \newcommand {\vnameref }[1]{\unskip ~\nameref {##1}\@vpageref [\unskip ]{##1}} \newcommand {\ref }[0]{\@ifstar \@refstar \T@ref } \newcommand {\pageref }[0]{\@ifstar \@pagerefstar \T@pageref } \newcommand {\nameref }[0]{\@ifstar \@namerefstar \T@nameref } \newcommand {\reserved@a }[2]{} \newcommand {\reserved@a }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{}$

Throughout this module, if something does not exist, write DNE in the answer box.

Recap Video

Take a look at the following video which recaps the ideas from the section.

Example Video

Below is a video showing a worked example.

Problems

A first-order differential equation is called autonomous if it has the form $\displaystyle \frac {dy}{dx} = F(y)$ for some function $F$ .

Which of the following differential equations are autonomous? Select all that apply.

$\displaystyle \frac {dy}{dt} = ty$ $\displaystyle \frac {dy}{dt} = y^2$ $\displaystyle \frac {dy}{dx} = x+y$ $\displaystyle \frac {dN}{dt} = N(1-N)$

Given a first order autonomous differential equation $\displaystyle \frac {dy}{dx} = F(y)$ , an equilibrium point $y_0$ is a point such that $F(y_0) = 0$ .

We can make use of a number line of sorts to help us classify these equilibrium points as stable, unstable, or semistable.

Consider $\displaystyle \frac {dy}{dx} = y(100-y)$ .

How many equilibrium points does the differential equation have? What are they?
The equilibrium points happen when $\displaystyle \frac {dy}{dx} = 0$ . This means $y(100-y) = 0,$ which gives two points, $y = 0$ and $y = \answer {100}$ .
Use a phase line to classify these equilibrium points.
To make a phase line, we will first make a vertical line representing $y$ and plot our equilibrium points.

What do the arrows represent? We will plug numbers in each region of the line into our differential equation. For example, if we plug in $y = 150$ into the equation, we get $\frac {dy}{dx}\Big |_{y=150} = 150(100-150),$ which is a number. Since $dy/dx < 0$ , this tells us $y$ is , hence the downward arrow in the region above $y = 100$ . Similarly, if we plug in $y=-50$ , we get $\frac {dy}{dx}\Big |_{y=-50} = -50(100-(-50)),$ which is a number. Since $dy/dx < 0$ , this tells us $y$ is , hence the downward arrow in the region below $y=0$ . Lastly, if $y = 50$ , we get $\frac {dy}{dx}\Big |_{y=50} = 50(100-50),$ which is a number. Since $dy/dx > 0$ , this tells us $y$ is , hence the upward arrow in the region between $y=0$ and $y=100$ .

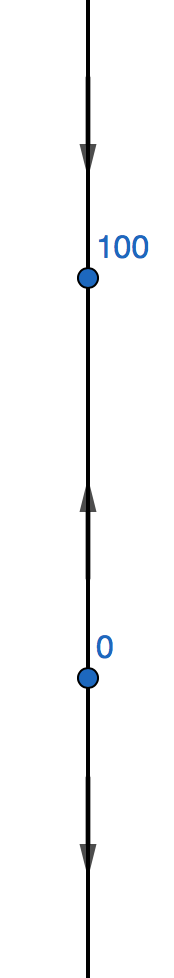
All this tells us that $y=100$ is a equilibrium point, and $y=0$ is a stableunstablesemistable equilibrium point.
If $y(0) = 125$ , what do you expect $\lim _{x \to \infty } y(x)$ to be?
Based on our classification of the equilibrium points from the previous part, we can say the limit will be $\answer {100}$ .

Consider $\displaystyle \frac {dy}{dt}= 2y^2-y^3+3y$ . How many equilibrium points does the differential equation have? $\answer {3}$ .

In increasing order, they are: $y = -1, \quad y = 0, \quad y = \answer {3}.$

The point $y=0$ is stableunstablesemistable , the point $y=-1$ is stableunstablesemistable , and the point $y=3$ is stableunstablesemistable .

An object is dropped with zero initial velocity. The vertical velocity (in ft/s) at time $t$ satisfies $\frac {dv}{dt} = -32 - 16v.$ How many equilibrium points does this differential equation have? $\answer {1}$ .

What is the only equilibrium point? $v = \answer {-2}$ .

Is this equilibrium point stable, unstable, or semistable?

stable unstable semistable

If $v(0) = 0$ , what do you expect $\lim _{t \to \infty } v(t)$ to be (assuming the object falls indefinitely)? $\answer {-2}$ .

We can also classify equilibrium points with a derivative test:

Suppose $y = y_0$ is an equilibrium point for $\displaystyle \frac {dy}{dx} = f(y)$ .

If $f'(y_0) > 0$ , then $y = y_0$ is an unstable equilibrium point.
If $f'(y_0) < 0$ , then $y = y_0$ is a stable equilibrium point.
If $f'(y_0) = 0$ , then the test is inconclusive, and you need to use the phase line approach.

Suppose $\displaystyle \frac {dy}{dx} = y^2(10-y)$ . How many equilibrium points does the differential equation have? $\answer {2}$ .

In increasing order, they are: $y=0,\quad y = \answer {10}.$

If $f(y) = y^2(10-y)$ , then $f'(y) = \answer {20y-3y^2}$ .

We have $f(0) = 0$ and $f'(10) = \answer {-100}$ .

Is $y = 10$ stable or unstable?

stable unstable semistable

Can the derivative test tell us anything about $y=0$ ?

Yes No

What does the phase line tell us about $y=0$ ? It is:

stable unstable semistable