4.3: Derivative Tests

$\newenvironment {prompt}{}{} \newcommand {\DeclareMathOperator }[2]{\@OldDeclareMathOperator {##1}{##2}\immediate \write \myfile {\unexpanded {\DeclareMathOperator }{\unexpanded {##1}}{\unexpanded {##2}}}} \newcommand {\reserved@a }[2]{} \newcommand {\HyperFirstAtBeginDocument }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{} \newcommand {\AppendGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Append \grfext@Check }{\grfext@Append \grfext@@Add }} \newcommand {\PrependGraphicsExtensions }[0]{\@ifundefined {Gin@extensions}{\let \Gin@extensions \@empty }{}\@ifstar {\grfext@Prepend \grfext@Check }{\grfext@Prepend \grfext@@Add }} \newcommand {\RemoveGraphicsExtensions }[1]{\@ifundefined {Gin@extensions}{\def \Gin@extensions {}}{\edef \grfext@tmp {\zap@space ##1 \@empty }\@for \grfext@ext :=\grfext@tmp \do {\def \grfext@next {\let \grfext@tmp \Gin@extensions \@expandtwoargs \@removeelement \grfext@ext \Gin@extensions \Gin@extensions \ifx \grfext@tmp \Gin@extensions \let \grfext@next \relax \fi \grfext@next }\grfext@next }}\grfext@Print \RemoveGraphicsExtensions } \newcommand {\epstopdfsetup }[0]{\setkeys {ETE}} \newcommand {\epstopdfcall }[1]{\ifETE@InsideSetfile \expandafter \@firstoftwo \else \expandafter \@secondoftwo \fi {‘##1}{\Gin@base \Gin@ext }} \newcommand {\epstopdfDeclareGraphicsRule }[4]{\ifx \\##4\\\@PackageError {epstopdf-base}{Conversion command is missing}\@ehc \else \begingroup \@ifundefined {Gin@rule@##1}{}{\@PackageInfo {epstopdf-base}{Redefining graphics rule for ‘##1’}}\endgroup \@namedef {Gin@rule@##1}####1{{##2}{##3}{\epstopdfcall {##4}}}\fi } \newcommand {\GetTitleStringSetup }[0]{\setkeys {gettitlestring}} \newcommand {\GetTitleString }[0]{\ifGTS@expand \expandafter \GetTitleStringExpand \else \expandafter \GetTitleStringNonExpand \fi } \newcommand {\GetTitleStringExpand }[1]{\def \GetTitleStringResult {##1}\begingroup \GTS@DisablePredefinedCmds \GTS@DisableHook \edef \x {\endgroup \noexpand \def \noexpand \GetTitleStringResult {\GetTitleStringResult }}\x } \newcommand {\GetTitleStringNonExpand }[1]{\def \GetTitleStringResult {##1}\global \let \GTS@GlobalString \GetTitleStringResult \begingroup \GTS@RemoveLeft \GTS@RemoveRight \endgroup \let \GetTitleStringResult \GTS@GlobalString } \newcommand {\GTS@DisableHook }[0]{} \newcommand {\label@hook }[0]{} \newcommand {\@currentlabelname }[0]{} \newcommand {\reserved@a }[0]{} \newcommand {\@safe@activestrue }[0]{} \newcommand {\@safe@activesfalse }[0]{} \newcommand {\nameref }[0]{\@ifstar \T@nameref \T@nameref } \newcommand {\Sectionformat }[2]{##1} \newcommand {\vnameref }[1]{\unskip ~\nameref {##1}\@vpageref [\unskip ]{##1}} \newcommand {\ref }[0]{\@ifstar \@refstar \T@ref } \newcommand {\pageref }[0]{\@ifstar \@pagerefstar \T@pageref } \newcommand {\nameref }[0]{\@ifstar \@namerefstar \T@nameref } \newcommand {\reserved@a }[2]{} \newcommand {\reserved@a }[0]{\AtBeginDocument } \newcommand {\reserved@a }[1]{} \newcommand {\reserved@a }[2]{}$

Throughout this module, if something does not exist, write DNE in the answer box.

Recap Video

Take a look at the following video which recaps the ideas from the section.

Example Video

Below is a video showing a worked example.

Problems

There’s a lot going on in this section, so let’s first have some questions to first test your understanding of the terminology and the tests.

If a differentiable function $f(x)$ satisfies $f'(x) > 0$ on an interval $I$ , then $f(x)$ is increasingdecreasingconcave upconcave down on the interval $I$ .

If a differentiable function $f(x)$ satisfies $f''(x) < 0$ on an interval $I$ , then $f(x)$ is increasingdecreasingconcave upconcave down on the interval $I$ .

If $x=c$ is a critical point of $f(x)$ and $f''(c) > 0$ , then which of the following is true?

There is a local maximum at $x=c$ . There is a local minimum at $x=c$ . There is neither a local maximum nor a local minimum at $x=c$ .

If $f(x)$ is infinitely differentiable and $f''(c) = 0$ , then it must be true that the graph of $y =f(x)$ has an inflection point at $x=c$ .

True False

Now for some longer problems.

Consider $f(x) = x^3-6x^2+3$ .

Find the intervals on which $f(x)$ is increasing/decreasing.
To do this, we will first find the critical points of $f(x)$ . The derivative of $f(x)$ is $f'(x) = \answer {3x^2-12x}.$ We will see where this is equal to $0$ or is undefined. There are no points where $f'(x)$ is undefined, so we will set it to $0$ instead. Solving $3x^2-12x = 0$ gives two points (list them in increasing order): $x = \answer {0},\quad x = \answer {4}.$ This breaks our number line up into pieces:

To figure out where $f(x)$ is increasing/decreasing, we plug in points in each region into $f'(x)$ . For example: $f'(-1) = \answer {15},\quad f'(1) = \answer {-9},\quad f'(5) = \answer {15}.$ This tells us $f(x)$ is increasing on which of the following intervals:
$(0,4)$ $(0,\infty )$ Only $(4,\infty )$ $(-\infty ,0)$ and $(4,\infty )$
We also know $f(x)$ is decreasing on which of the following intervals?
Only $(0,4)$ $(0,\infty )$ Only $(4,\infty )$ $(-\infty ,0)$ and $(4,\infty )$
Find and classify all local extrema of $f(x)$ .
From the previous part, we know there are two critical points: $x=0$ and $x=4$ . We also know from our increasing/decreasing analysis that there is a local at $x=0$ and there is a local at $x=4$ .
Find the intervals on which $f(x)$ is concave up or concave down.
We now need to examine $f''(x)$ . Taking the second derivative, we find $f''(x) = \answer {6x-12}.$ This isn’t undefined anywhere, so we set it to $0$ . This gives us one point: $x = \answer {2}$ . This splits our number line into two pieces:

We can plug in a number in each region into the second derivative: $f''(1) = \answer {-6},\quad f''(3) = \answer {6}.$ This tells us $f(x)$ is concave up on which of the following intervals?
$(2,\infty )$ $(-\infty ,\infty )$ $(-\infty ,2)$
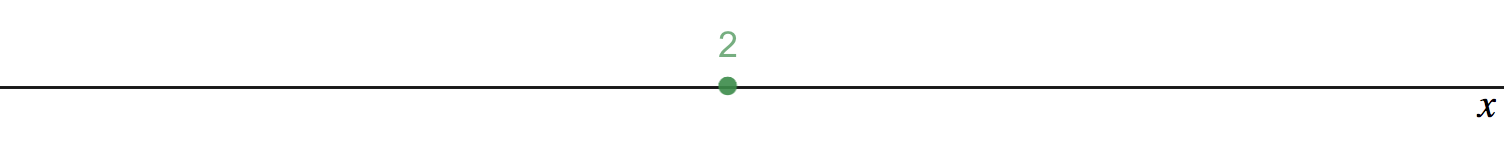We also know $f(x)$ is concave down on which of the following intervals?
$(2,\infty )$ $(-\infty ,\infty )$ $(-\infty ,2)$
Find all inflection points of $f(x)$ .
These are points where $f(x)$ changes concavity. From the previous part, we know there is one inflection point at $x=\answer {2}$ .

How would we use the second derivative test to classify the critical points from the previous problem?

We found two critical points: $x=0$ and $x=4$ . To classify them, we plug them into $f''(x) = 6x-12$ . We get $f''(0) = \answer {-12},\quad f''(4) = \answer {12}.$ This tells us $f(x)$ is concave updown at $x=0$ , so $f(x)$ has a local maxmin at $x=0$ . We also get that $f(x)$ is concave updown at $x=4$ , so $f(x)$ has a local maxmin at $x=4$ .

Consider $f(x) = x^3+24x^2$ . How many critical points does $f(x)$ have? $\answer {2}$ .

The critical points happen at $x=0$ and $x = \answer {-16}$ .

Which of the following describe the intervals on which $f(x)$ is increasing?

Only $(-\infty ,-16)$ $(-\infty ,-16)$ and $(0,\infty )$ Only $(0,\infty )$ Only $(-16,0)$

There is a local maximumminimum at $x=-16$ .

There is a local maximumminimum at $x=0$ .

How many inflection points does $f(x)$ have? $\answer {1}$ .

The inflection point happens at $x=\answer {-8}$ .

Which of the following describe all intervals on which $f(x)$ is concave up?

$(-\infty ,-8)$ $(-8,\infty )$ $(0,\infty )$

Which of the following describe all intervals on which $f(x)$ is concave down?

$(-\infty ,-8)$ $(-8,\infty )$ $(0,\infty )$

Consider $f(x) = 2x+\cos (2x)$ on the interval $(0,\pi )$ . How many critical points does $f(x)$ have? $\answer {2}$ .

The critical point happens at $x = \answer {\pi /4}$ .

Which of the following describe the intervals on which $f(x)$ is increasing?

Only $(0,\pi /4)$ $(0,\pi /4)$ and $(\pi /4,\pi )$ Only $(\pi /4,\pi )$ Only $(0,\pi /2)$

Which of the following is true at $x=\pi /4$ ?

There is a local maximum There is a local minimum There is neither a local maximum nor a local minimum

How many inflection points does $f(x)$ have? $\answer {2}$ .

The inflection points happen at $x=\pi /4$ and $x= \answer {3\pi /4}$ .

Which of the following describe all intervals on which $f(x)$ is concave up?

Only $(\pi /4,3\pi /4)$ $(0,\pi /4)$ and $(3\pi /4,\pi )$ Only $(\pi /4,\pi )$

Which of the following describe all intervals on which $f(x)$ is concave down?

Only $(\pi /4,3\pi /4)$ $(0,\pi /4)$ and $(3\pi /4,\pi )$ Only $(\pi /4,\pi )$